So the exam was over on Monday. I am still not sure if I will need to do an S/U on the module, but if I ever get so much as a B+ I would be content and hell I'll drag Surya out to one of those green spaces left around school and dance around with him in joy. (FYI: Surya is the other guy from Engineering who took this module for fun.)
Artist's Impression
Bidding for this module was foolhardiness from the start. Learning the methods of Real Analysis has been an uphill task, but explaining its contents to interested passers-by was also difficult, so I'll do just that.
The Meaning of Real Analysis
Calculus, the crown jewels of MA1505, was invented independently by different folks in the 14th to 17th centuries. Apparently where caluclus started, it was mathematically sloppy, but got away with it for being so useful. Nonetheless it was met with attacks from other scholars, among them my old friend Bishop Berkeley. In time, the properties of the calculus-universe would be gradually built up and moulded by those willing to clean up Newton's and Leibniz's mess, and the cleaning process became the field we call Real Analysis.
Formalities: Definition, Theorems and Proofs
Because it looks base-wards from calculus, rather than to the frontiers of math, most of the concepts we tackle are simple and intuitive. This sadly does not make them any easier. It does, however, mean that we have to
scrutinise our every preconception to all that we think we know to be true. This is achieved by definitions made precise with numerical gauges and basic rules of algebra. A case in point:
Continuity of a function is
MA1505: "that you can draw its graph in one go without having to lift your pencil from the paper."
MA2108: "if ∀ε>0 ∃δ>0 such that ∣x-a∣<δ ⇒ ∣f(x)-f(a)∣<ε, then f is continuous on a."
So instead of drawing a continuous line without lifting the pencil from the paper to prove a point, you broke everything up into numbers and shifted them around and prayed hard that they agreed with you.
This may seem frivolous at first, but as the lectures go on we saw the definition spew out quite some interesting conclusions. For example, a function can be continuous
at only one point. Trying to show it with pencil and paper is quite pointless, but you will be able to show, with definition, that something like
f(x) = {x2 if x is rational, 0 if x is irrational}
does just that at x=0.
Also I mentioned Thomae's Popcorn function some entries back. You guys totally should look at it again. The idiot is continuous on all irrational x and discontinuous on all rational x. It is so crazy I still find it hard to swallow, even if I am able to prove it back to front.
The start of the module was the hardest because we were having our hands tied up behind out backs and mulling over things like a+b = b+a like we were newborns. Things got better later on when we found out certain theorems have been proven so that we can use them with impunity. But then there were other things to worry about.
Aesthetics: Niceness
This is to recall the things I picked up last semester about mathematicians being artists inside. William Byers wrote about mathematical beauty. David Hilbert complained about finding not enough imagination in his students. Leung Man Chun played the Concierto de Aranjuez before every lecture.
Artists would be familiar to the fact that mathematics can be, and are often used to make art. In particular, I have come across a section of the shelf on Medical/Science library (5th floor) with material on Splines, NURBs and other cool inscrutable stuff we find mentioned and used all the time in 3D modeling software. Apparently meshSmooth, spline curves, and modeling by contour uses Real Analysis. This is so interesting
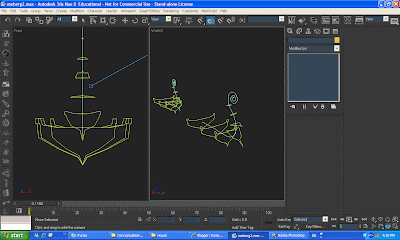
I would like to take this opportunity to show off my "modeling by contour"
In Real Analysis,
niceness is a real thing, and is mathematically defined. A continuous function is a nice mapping of ℝ on ℝ, and a uniformly continuous function is a nice continuous function. And a properly divergent sequence diverges quite neatly also. And together they bask your notes in a warm glow.
How to prepare for this module
Being a Math student helps. Having taken MA1100 Fundamentals of Mathematics also helps, because your teachers will assume you picked up from there. If you're one of my ilk, it helps to understand that this is no maths that you have seen before. It is useless to think of anything as "plug in formulas to get a number" (even though I have guiltily indulged in "plug in theorems to get a proof" from time to time). It is helpful to remind yourself that
nothing is static,
everything is uncertain, and that
skepticism is the rule before where the fatal QED lies. Having a lively mind helps, so taking up some sport would be a good idea.
Postscript
Exam Qn 3 b) iii)
Ohmigod, look at this guy, just look at him.
